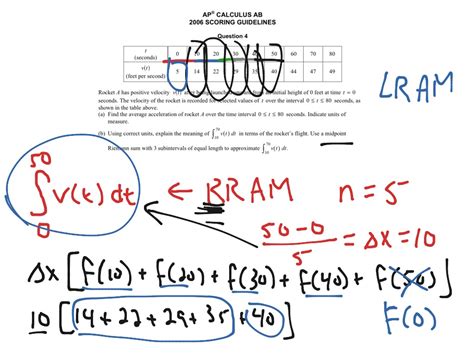
Introduction
The midpoint Riemann sum is a fundamental technique in calculus used to approximate the definite integral of a function. It involves dividing the area under the curve into smaller rectangles and summing their areas. This article provides a comprehensive guide to the midpoint Riemann sum, including its definition, formula, applications, and a detailed table for quick reference.
Definition and Formula
The midpoint Riemann sum is defined as:
∫[a, b] f(x) dx ≈ Δx [f(x1/2) + f(x3/2) + ... + f(xn-1/2)]
where:
- Δx = (b – a) / n is the width of each rectangle
- n is the number of subintervals
- x1/2, x3/2, …, xn-1/2 are the midpoints of each subinterval
Applications
The midpoint Riemann sum finds extensive use in various applications, including:
- Numerical integration: Approximating the area under the curve of a function
- Physics: Calculating force, work, and other physical quantities
- Economics: Estimating consumer surplus and producer surplus
Table of Midpoint Riemann Sums
For quick reference, the following table summarizes the formula and key information about the midpoint Riemann sum:
| Property | Value |
|---|---|
| Formula | ∫[a, b] f(x) dx ≈ Δx [f(x1/2) + f(x3/2) + … + f(xn-1/2)] |
| Error bound | |
| Advantages | Provides a more accurate approximation than the left or right Riemann sum |
| Disadvantages | Requires more calculations than the left or right Riemann sum |
Error Analysis
The error bound formula for the midpoint Riemann sum is:
|∫[a, b] f(x) dx - M(b - a)^2| / 24n^2
The error decreases as the number of subintervals, n, increases.
Tips and Tricks
- Choose a large enough n to ensure accuracy, but avoid making n excessively large, as this can lead to numerical instability.
- Use a calculator or computer program to perform the calculations, as manual computation can be tedious.
- Double-check your calculations to minimize errors.
- Consider using other numerical integration techniques, such as the trapezoidal rule or Simpson’s rule, for higher accuracy.
Pain Points and Motivations
Pain Points:
- The midpoint Riemann sum can be time-consuming to compute manually.
- It can be difficult to determine the optimal number of subintervals to use.
- The error bound can be large, especially for functions with high curvature.
Motivations:
- Despite its limitations, the midpoint Riemann sum remains a valuable tool for approximating integrals.
- It provides a more accurate approximation than the left or right Riemann sum.
- It can be used to estimate the area under the curve of functions that are not easily integrated analytically.
Effective Strategies
Strategies to Address Pain Points:
- Use technology to automate the computation process.
- Experiment with different values of n to find the optimal trade-off between accuracy and efficiency.
- Consider using alternative numerical integration techniques for improved accuracy.
Strategies to Leverage Motivations:
- Apply the midpoint Riemann sum to estimate integrals of functions that are difficult to integrate analytically.
- Utilize the midpoint Riemann sum to calculate physical quantities in physics, such as work and force.
- Employ the midpoint Riemann sum in economic applications, such as estimating consumer and producer surplus.
Conclusion
The midpoint Riemann sum is a fundamental technique in calculus for approximating the definite integral of a function. This article has provided a comprehensive overview of its definition, formula, applications, error analysis, tips, and tricks. By understanding the midpoint Riemann sum, students can effectively approximate integrals and solve a wide range of problems in calculus and beyond.