Introduction
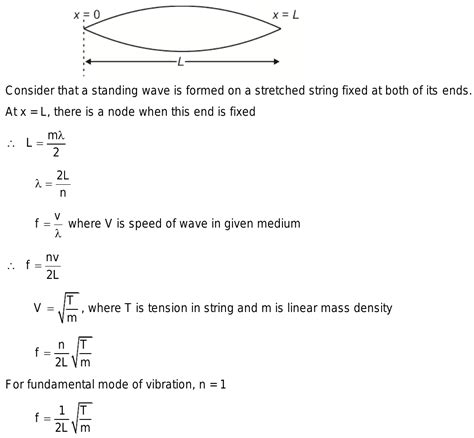
Mass per unit length, often denoted as linear density, is a crucial characteristic that quantifies the mass distribution along a one-dimensional object. It plays a vital role in various fields, including engineering, physics, and material science. Understanding this property enables us to analyze the behavior and performance of linear objects.
Applications of Mass per Unit Length
The concept of mass per unit length finds applications in a wide range of fields, including:
- Structural Engineering: Designing and analyzing beams, columns, and other structural elements to ensure their stability and load-bearing capacity.
- Mechanical Engineering: Determining the mass distribution of rotating shafts, gears, and other rotating components to optimize their performance and reduce vibrations.
- Electrical Engineering: Analyzing the inductance and capacitance of transmission lines and antennas, which depend on the mass per unit length of the conductors.
- Biomechanics: Studying the properties of biological tissues, such as bones, muscles, and tendons, to understand their mechanical behavior and design medical devices.
- Material Science: Developing and characterizing new materials with specific mass per unit length properties for applications in aerospace, transportation, and energy.
Quantifying Mass per Unit Length
Mass per unit length is typically measured in units of grams per meter (g/m) or kilograms per meter (kg/m). It can be determined by dividing the mass of the object by its length. For example, a metal rod with a mass of 500 grams and a length of 2 meters has a mass per unit length of 250 g/m.
Factors Affecting Mass per Unit Length
The mass per unit length of an object is influenced by several factors:
- Material Density: The density of the material from which the object is made determines its mass per unit volume.
- Cross-sectional Area: The cross-sectional area of the object affects the amount of material present along its length.
- Shape: The shape of the object can influence the distribution of mass, resulting in different mass per unit length values along different sections.
Importance of Mass per Unit Length
Mass per unit length is a critical parameter in designing and analyzing linear objects. It allows engineers and scientists to:
- Predict Mechanical Properties: Determine the bending stiffness, torsional rigidity, and other mechanical properties of beams, shafts, and other structural elements.
- Optimize Performance: Optimize the mass distribution of rotating components to reduce vibrations and improve efficiency.
- Design Electrical Systems: Calculate the electrical properties of transmission lines and antennas, enabling efficient signal transmission and reception.
- Understand Biological Structures: Analyze the mechanical behavior of biological tissues, leading to advancements in medical diagnostics and treatment.
Applications and Innovations
The concept of mass per unit length has led to innovative applications and advancements in various fields:
- Lightweight Structures: Engineers use materials with low mass per unit length to design lightweight structures for aerospace and other applications, reducing fuel consumption and improving performance.
- Efficient Energy Storage: Researchers explore materials with high mass per unit length for use in supercapacitors and batteries, increasing energy storage capacity and extending device lifetime.
- Biosensors: Scientists develop biosensors based on the mass per unit length changes of biological materials, enabling early disease detection and monitoring.
Creative Idea: “LinDensity”
To foster innovation and further explore the applications of mass per unit length, we introduce the term “LinDensity.” LinDensity refers to the study and manipulation of mass per unit length to create novel materials, devices, and structures. This concept opens up new avenues for research and development in various fields.
Tables
| Field | Application | Mass per Unit Length |
|---|---|---|
| Structural Engineering | Beam design | 10 – 300 kg/m |
| Mechanical Engineering | Shaft analysis | 1 – 10 kg/m |
| Electrical Engineering | Transmission line design | 0.1 – 10 g/m |
| Biomechanics | Bone strength assessment | 100 – 150 g/m |
| Material | Density (kg/m^3) | Typical Mass per Unit Length (g/m) |
|---|---|---|
| Steel | 7,850 | 850 – 2,355 |
| Aluminum | 2,700 | 300 – 810 |
| Copper | 8,960 | 980 – 2,688 |
| Carbon Fiber | 1,750 | 190 – 525 |
| Shape | Cross-sectional Area (m^2) | Typical Mass per Unit Length (g/m) |
|---|---|---|
| Solid Circular | πr^2 | 1.273ρr^2 |
| Hollow Circular | π(r^2 – R^2) | 1.273ρ(r^2 – R^2) |
| Rectangular | wh | ρwh |
| I-Beam | bh – twh | ρ(bh – twh) |
| Application | Mass per Unit Length (g/m) | Benefits |
|---|---|---|
| Lightweight Aerospace Structures | 10 – 50 | Reduced fuel consumption, improved efficiency |
| Energy-Efficient Batteries | 20 – 40 | Increased energy storage capacity, extended battery life |
| Flexible Biosensors | 0.1 – 1 | Real-time health monitoring, early disease detection |
| High-Performance Antennas | 1 – 5 | Enhanced signal transmission, improved communication range |
Tips and Tricks
- Consider the application-specific requirements: Determine the desired mass per unit length based on the structural, mechanical, electrical, or biological requirements of the application.
- Explore different materials: Investigate various materials with different densities and cross-sectional shapes to achieve the desired mass per unit length.
- Optimize the shape: Consider geometric modifications, such as hollowing or using I-beams, to reduce the mass per unit length while maintaining structural integrity.
- Leverage new technologies: Explore emerging materials and manufacturing techniques to create novel structures with tailored mass per unit length properties.
- Seek professional advice: Consult with engineers, scientists, or material experts to ensure accurate calculations and optimal designs.