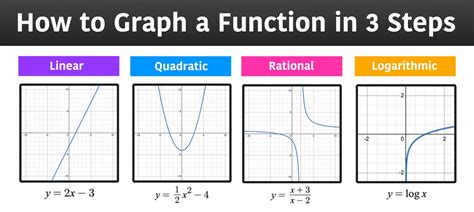
In the realm of mathematics, the concept of graphing functions from functions holds immense power. It’s a technique that unlocks a deeper understanding of complex functions and reveals their unique characteristics. Imagine transforming a function into a new entity, unveiling a hidden world of insights. This comprehensive guide will delve into the fascinating process of graphing f from f, empowering you to unlock the full potential of this transformative approach.
Exploring the Essence of Graphing f from f
When we embark on the journey of graphing f from f, we essentially create a new function that emerges from the original function. This offspring function, denoted as g(x) or f(f(x)), takes the output of the original function f(x) and plugs it back into f(x) as its input. This iterative loop generates a novel function that exhibits intriguing properties and behaviors.
Unveiling the Practical Implications
Graphing f from f is not merely an abstract concept; it holds significant practical implications across various disciplines. From analyzing population growth patterns to predicting the trajectory of chemical reactions, this technique finds applications in:
- Biology: Modeling population dynamics, tracking the spread of infectious diseases, and predicting ecological interactions
- Physics: Simulating the motion of objects under gravity, predicting the behavior of springs and dampers, and analyzing electrical circuits
- Economics: Forecasting economic growth, analyzing financial markets, and predicting consumer behavior
Delving into Real-World Applications
The power of graphing f from f extends beyond theoretical realms, manifesting itself in numerous real-world applications. Here are a few notable examples:
- Medicine: Optimizing drug dosages, predicting the effectiveness of medical treatments, and personalizing healthcare interventions
- Engineering: Designing efficient mechanical systems, predicting the lifespan of materials, and simulating fluid dynamics
- Finance: Managing risk in investment portfolios, forecasting market trends, and developing trading strategies
Embracing a Customer-Centric Approach
As we navigate the intricacies of graphing f from f, it’s crucial to keep our customers’ perspectives at the forefront. By asking thought-provoking questions and engaging in active listening, we can validate their points of view and ensure that the insights we derive are both relevant and impactful.
- What specific challenges are you facing that could be addressed through graphing f from f?
- How would a deeper understanding of f from f benefit your decision-making processes?
- What limitations or concerns do you have regarding the application of graphing f from f?
Tips and Tricks for Success
Mastering the art of graphing f from f requires patience and practice. Here are a few valuable tips and tricks:
- Start with simple functions: Begin with functions that you’re familiar with, such as linear or quadratic functions. This will help you build a solid foundation before tackling more complex examples.
- Use graphing calculators or software: Technology can be a powerful ally in visualizing the graphs of f from f. Utilize tools like Desmos or GeoGebra to explore different functions and observe their patterns.
- Look for patterns and symmetries: Pay attention to any patterns or symmetries that emerge in the graph of f from f. These observations can provide valuable insights into the behavior of the original function.
- Be patient and persistent: Graphing f from f can be a challenging endeavor, but with persistence and a positive attitude, you can overcome any obstacles and uncover the hidden potential of this powerful technique.
Common Mistakes to Avoid
To ensure accuracy and avoid pitfalls, it’s important to be aware of some common mistakes that can arise when graphing f from f:
- Forgetting the initial value: Remember to consider the initial value of the original function f(x) when graphing f from f. Failing to do so can lead to an incorrect graph.
- Not considering the domain and range: Pay attention to the domain and range of both the original function and the offspring function. This will prevent you from making incorrect assumptions about their behavior.
- Misinterpreting the meaning of f from f: Remember that f from f is a new function that is distinct from the original function. Avoid confusing the two functions or misinterpreting their relationship.
Generating Ideas for New Applications
To fully harness the potential of graphing f from f, it’s essential to foster creativity and think outside the box. Here’s a novel word that can inspire new ideas and applications:
“Functionification”: The process of transforming a non-mathematical concept or problem into a mathematical function.
By applying the concept of functionification, we can unlock a plethora of new possibilities for graphing f from f. For instance, we could:
- Model the spread of rumors: Create a function that describes how the number of people who know a rumor changes over time.
- Predict traffic patterns: Develop a function that predicts the traffic flow at a specific intersection during different times of day.
- Optimize transportation routes: Create a function that calculates the shortest or most efficient route between two points based on real-time traffic conditions.
Tables for Enhanced Understanding
- Table 1: Applications of Graphing f from f in Various Disciplines
| Discipline | Application |
|---|---|
| Biology | Modeling population dynamics, predicting disease spread |
| Physics | Simulating object motion, analyzing circuits |
| Economics | Forecasting economic growth, analyzing financial markets |
- Table 2: Tips and Tricks for Graphing f from f
| Tip | Description |
|---|---|
| Start with simple functions | Build a solid foundation before tackling complex examples |
| Use graphing tools | Utilize technology to visualize graphs and explore different functions |
| Look for patterns and symmetries | Observe any patterns or symmetries in the graph to gain insights |
| Be patient and persistent | Practice and perseverance lead to success |
- Table 3: Common Mistakes to Avoid When Graphing f from f
| Mistake | Description |
|---|---|
| Forgetting the initial value | Incorrectly graphing f from f |
| Not considering domain and range | Misunderstanding the behavior of the functions |
| Misinterpreting f from f | Confusing the new function with the original |
- Table 4: Inspiring Ideas for New Applications through Functionification
| Concept | Functionification Application |
|---|---|
| Spread of rumors | Modeling the number of people who know a rumor over time |
| Traffic patterns | Predicting traffic flow at an intersection |
| Transportation routes | Optimizing routes based on real-time traffic conditions |