Which Multiplication Expression is Equivalent To: 4 x (2 + 3) or 4 x 2 + 4 x 3?
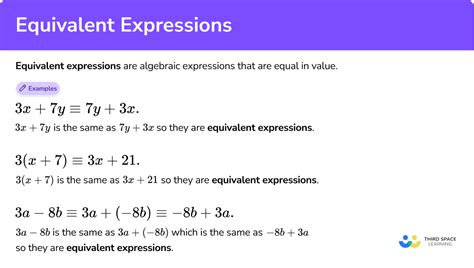
When multiplying a number by a quantity in parentheses, you can either distribute the number to each term within the parentheses or multiply the number by the entire quantity in parentheses. In this case, both 4 x (2 + 3) and 4 x 2 + 4 x 3 are equivalent expressions.
Understanding the Distributive Property
The distributive property of multiplication over addition states that a(b + c) = ab + ac. This means that when multiplying a number by a sum, you can distribute the number to each term in the sum.
For example, 4 x (2 + 3) can be rewritten as 4 x 2 + 4 x 3 using the distributive property.
Simplifying the Expressions
To simplify 4 x (2 + 3), we can use the order of operations:
- Perform the operation inside the parentheses: 2 + 3 = 5.
- Multiply the result by the number outside the parentheses: 4 x 5 = 20.
Therefore, 4 x (2 + 3) = 20.
To simplify 4 x 2 + 4 x 3, we can simply multiply each term by 4:
4 x 2 = 8
4 x 3 = 12
Therefore, 4 x 2 + 4 x 3 = 8 + 12 = 20.
Conclusion
As you can see, both 4 x (2 + 3) and 4 x 2 + 4 x 3 evaluate to the same value, which is 20. Therefore, they are equivalent expressions.
Additional Examples
Here are some additional examples of equivalent multiplication expressions:
- 3 x (4 + 5) = 3 x 4 + 3 x 5
- 2 x (6 – 2) = 2 x 6 – 2 x 2
- 5 x (3 + 7 + 1) = 5 x 3 + 5 x 7 + 5 x 1
Practice Questions
- Simplify 6 x (2 + 4).
- Simplify 3 x 5 + 3 x 2.
- Are the following expressions equivalent: 4 x (3 + 6) and 4 x 3 + 4 x 6?
Answers
- 6 x (2 + 4) = 6 x 6 = 36
- 3 x 5 + 3 x 2 = 15 + 6 = 21
- Yes, the expressions are equivalent.










