Pain Points

Students preparing for the New York State Regents Exam in Algebra 1 often struggle to memorize and apply the numerous formulas required for success. This formula sheet provides a comprehensive resource for students to reference during the exam, reducing stress and increasing their chances of a high score.
Motivations
The Regents Exam is a key assessment for high school students in New York State, and a strong performance can open doors to future opportunities. By providing a concise and accessible formula sheet, we empower students to approach the exam with confidence and focus on demonstrating their problem-solving skills.
Why it Matters
Algebra 1 is the foundation for higher-level mathematics courses, and mastering the fundamental concepts and formulas is essential for future success in STEM fields. A comprehensive formula sheet empowers students to retain information and apply it to real-world problems, fostering a deeper understanding of mathematics and its practical applications.
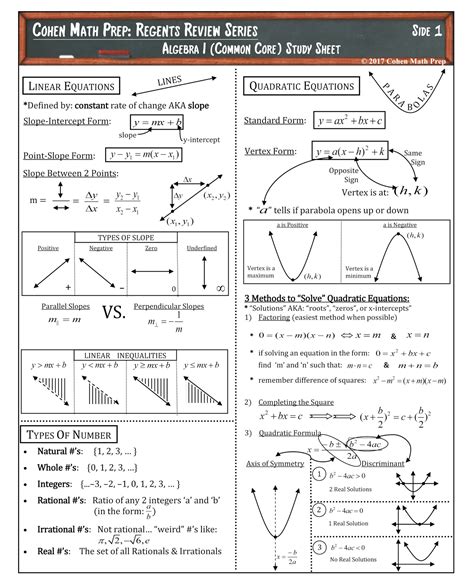
Linear Equations and Inequalities
- Slope-Intercept Form: y = mx + b
- Point-Slope Form: y – y1 = m(x – x1)
- Standard Form: Ax + By = C
- Solving Equations: x = (C – By) / A
- Solving Inequalities: Plot the boundary line and shade the appropriate half-plane
Exponents and Polynomials
- Exponents: am * an = am+n, (am)n = amn
- Polynomials: Standard form: axn + bxn-1 + … + c
- Degree: The highest exponent of the variable in the polynomial
- Factoring: Find pairs of factors that multiply to give the coefficients and add to give the constant term
Graphing
- Slope: m = (y2 – y1) / (x2 – x1)
- Y-intercept: (0, b)
- Vertex: (h, k), where h = -b/2a and k = -D/4a (D is the discriminant)
- Axis of Symmetry: x = h
Systems of Equations
- Substitution: Solve one equation for a variable and substitute into the other equation
- Elimination: Add or subtract the equations to eliminate a variable
Inequalities
- Compound Inequalities: Plot the boundary lines and shade the appropriate region
- Absolute Value Inequalities: |x| < a => -a < x < a, |x| > a => x < -a or x > a
Matrices
- Matrix: A rectangular array of numbers arranged in rows and columns
- Determinant (2×2 matrix): |A| = ad – bc
- Inverse (2×2 matrix): A-1 = 1/|A| * [d -b-a c]
Pythagorean Theorem and Trigonometry
- Pythagorean Theorem: a2 + b2 = c2
-
Trigonometric Ratios:
- Sine: sin(θ) = opposite / hypotenuse
- Cosine: cos(θ) = adjacent / hypotenuse
- Tangent: tan(θ) = opposite / adjacent
Additional Formulae
- Quadratic Formula: x = (-b ± √(b2 – 4ac)) / 2a
- Circumference of a Circle: C = 2πr
- Area of a Circle: A = πr2
- Volume of a Sphere: V = (4/3)πr3
Table 1: Linear Equations and Inequalities
| Equation/Inequality | Form | Solution |
|---|---|---|
| Slope-Intercept Form | y = mx + b | x = (C – By) / A |
| Point-Slope Form | y – y1 = m(x – x1) | None |
| Standard Form | Ax + By = C | x = (C – By) / A |
| Linear Inequality | Ax + By > C | Plot and shade above the boundary line |
| Linear Inequality | Ax + By < C | Plot and shade below the boundary line |
Table 2: Exponents and Polynomials
| Formula | Description | Example |
|---|---|---|
| am * an = am+n | Multiplying exponents | 23 * 25 = 28 |
| (am)n = amn | Raising a power to a power | (32)3 = 36 |
| axn + bxn-1 + … + c | Standard form of a polynomial | 2x3 + 5x2 – 7x + 1 |
| Degree | Highest exponent in a polynomial | 3 (for 2x3 + 5x2 – 7x + 1) |
Table 3: Graphing
| Formula | Description | Example |
|---|---|---|
| Slope | (y2 – y1) / (x2 – x1) | (3 – 1) / (2 – 1) = 2 |
| Y-intercept | (0, b) | (0, 5) |
| Vertex | (h, k), where h = -b/2a and k = -D/4a | (-1, 2) |
| Axis of Symmetry | x = h | x = -1 |
Table 4: Matrices
| Formula | Description | Example |
|---|---|---|
| Determinant (2×2 matrix) | A | |
| Inverse (2×2 matrix) | A-1 = 1/ | A |
FAQs
-
When should I use the formula sheet?
- During the Regents Exam, use the formula sheet as a quick reference for formulas and concepts.
-
What formulas are not included on the formula sheet?
- Some advanced or rarely used formulas are not included. Refer to your textbook or teacher for additional support.
-
How can I maximize the benefit of the formula sheet?
- Review the formulas before the exam and make sure you understand how to apply them.
- Practice using the formulas to solve problems to ensure proficiency.
-
Can I use the formula sheet on all parts of the Regents Exam?
- Yes, the formula sheet can be used for all questions on the Regents Exam in Algebra 1.
-
Is the formula sheet available online?
- Yes, the formula sheet is typically provided by the New York State Education Department and can be found on their website or in official exam preparation materials.
-
How long is the Regents Exam in Algebra 1?
- The Regents Exam in Algebra 1 is typically 3 hours long.
-
What score do I need to pass the Regents Exam in Algebra 1?
- A score of 65 or higher is required to pass the Regents Exam in Algebra 1.
-
What resources are available to help me prepare for the Regents Exam in Algebra 1?
- Utilize textbooks, review materials, online practice tests, and seek guidance from teachers or tutors.