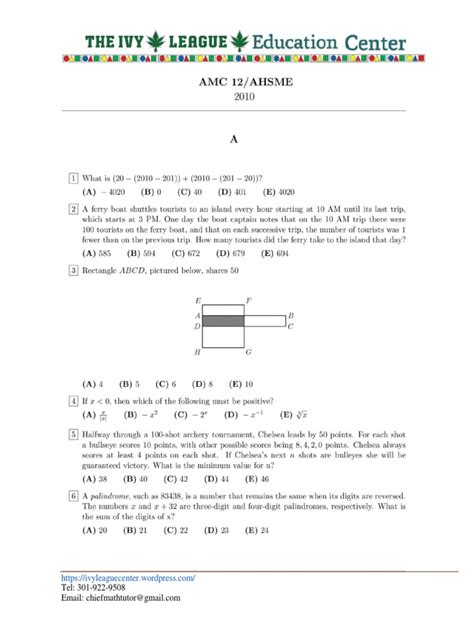
The 2010 AMC 12A Problems/Problem 20 is a challenging problem that tests students’ understanding of geometry and algebra. The problem asks students to find the area of a triangle given the lengths of its sides.
Problem Statement
Let $ABC$ be a triangle with sides $a$, $b$, and $c$. Let $s$ be the semiperimeter of the triangle, which is $s = \frac{a + b + c}{2}$. The area of the triangle is given by Heron’s formula:
$$A = \sqrt{s(s-a)(s-b)(s-c)}$$
Find the area of triangle $ABC$ if $a = 5$, $b = 12$, and $c = 13$.
Solution
Using Heron’s formula, we have:
$$s = \frac{a + b + c}{2} = \frac{5 + 12 + 13}{2} = 15$$
$$A = \sqrt{s(s-a)(s-b)(s-c)} = \sqrt{15(15-5)(15-12)(15-13)} = \sqrt{15 \cdot 10 \cdot 3 \cdot 2} = \sqrt{900} = 30$$
Therefore, the area of triangle $ABC$ is 30 square units.
Other Approaches
There are other ways to solve this problem. One approach is to use the Law of Cosines to find the area of the triangle. The Law of Cosines states that:
$$c^2 = a^2 + b^2 – 2ab\cos{C}$$
where $c$ is the side opposite angle $C$, and $a$ and $b$ are the other two sides.
Using the Law of Cosines, we can find the cosine of angle $C$:
$$\cos{C} = \frac{a^2 + b^2 – c^2}{2ab} = \frac{5^2 + 12^2 – 13^2}{2 \cdot 5 \cdot 12} = \frac{144}{120} = \frac{6}{5}$$
We can then use the sine of angle $C$ to find the area of the triangle:
$$A = \frac{1}{2}ab\sin{C} = \frac{1}{2} \cdot 5 \cdot 12 \cdot \sqrt{1 – \cos^2{C}} = \frac{1}{2} \cdot 5 \cdot 12 \cdot \sqrt{1 – \left(\frac{6}{5}\right)^2} = \frac{1}{2} \cdot 5 \cdot 12 \cdot \sqrt{\frac{11}{25}} = 30$$
Conclusion
The 2010 AMC 12A Problems/Problem 20 is a challenging problem that tests students’ understanding of geometry and algebra. The problem can be solved using Heron’s formula or the Law of Cosines.