In mathematics, integration is a fundamental operation used to find the area under a curve or the volume of a solid. In some cases, it is necessary to change the order of integration to simplify the calculation.

When to Change the Order of Integration
The order of integration can be changed when the integral is defined over a region that can be expressed as a product of two intervals. For example, the integral of $f(x, y)$ over the region $R = [a, b] \times [c, d]$ can be written as
$$\int_a^b \int_c^d f(x, y) dy dx$$
or
$$\int_c^d \int_a^b f(x, y) dx dy.$$
The order of integration can be changed if the limits of integration for one of the variables can be expressed as functions of the other variable. For example, if $R$ is the region bounded by the curves $y = x$ and $y = x^2$, then the integral of $f(x, y)$ over $R$ can be written as
$$\int_0^1 \int_x^{x^2} f(x, y) dy dx$$
or
$$\int_0^1 \int_0^y f(x, y) dx dy.$$
How to Change the Order of Integration
To change the order of integration, the following steps must be followed:
- Write the integral with the original order of integration.
- Determine the limits of integration for the new order of integration.
- Rewrite the integral with the new limits of integration.
Example
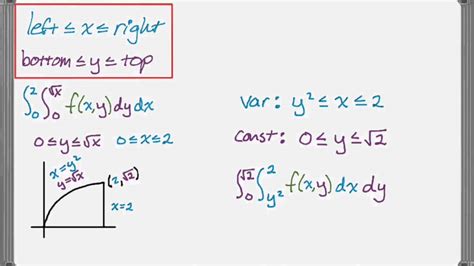
Consider the integral
$$\int_0^1 \int_x^{x^2} f(x, y) dy dx.$$
To change the order of integration, the following steps are used:
- Write the integral with the original order of integration:
$$\int_0^1 \int_x^{x^2} f(x, y) dy dx.$$
-
Determine the limits of integration for the new order of integration. The limits of integration for $x$ are $0$ and $1$, and the limits of integration for $y$ are $0$ and $\sqrt{x}$.
-
Rewrite the integral with the new limits of integration:
$$\int_0^1 \int_0^{\sqrt{x}} f(x, y) dx dy.$$
Applications of Changing the Order of Integration
Changing the order of integration can be useful in a variety of applications, including:
- Finding the volume of a solid
- Calculating the area under a curve
- Evaluating integrals in polar coordinates
- Solving differential equations
Conclusion
Changing the order of integration is a powerful technique that can be used to simplify the calculation of integrals. By following the steps outlined in this article, you can easily change the order of integration and solve a variety of problems.