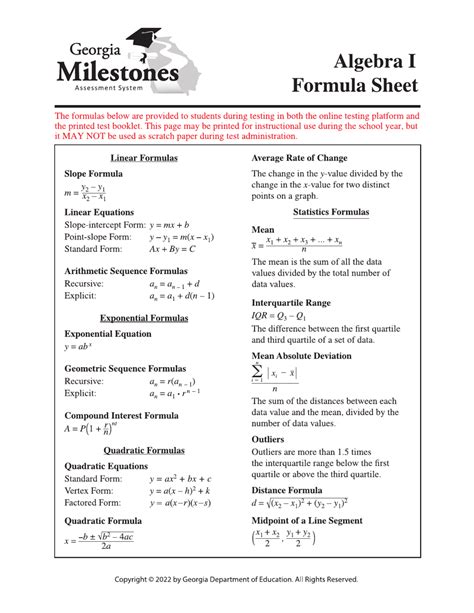
Essential Formulas
Linear Equations
- Slope-intercept form: y = mx + b
- Point-slope form: y – y1 = m(x – x1)
- Standard form: Ax + By = C
- Slope of a line: m = (y2 – y1) / (x2 – x1)
- Distance between two points: d = sqrt((x2 – x1)^2 + (y2 – y1)^2)
Polynomials
- Quadratic formula: x = (-b ± sqrt(b^2 – 4ac)) / 2a
- Binomial theorem: (a + b)^n = Σ[k=0 to n] (n choose k) a^(n-k)b^k
- Remainder theorem: r = p(c) when x – c is a factor of p(x)
- Factor theorem: If p(c) = 0, then x – c is a factor of p(x)
- Difference of squares factorization: a^2 – b^2 = (a + b)(a – b)
Exponents and Radicals
- Product rule: a^m * a^n = a^(m + n)
- Quotient rule: a^m / a^n = a^(m – n)
- Power of a power rule: (a^m)^n = a^(mn)
- Root rule: sqrt(a^m) = a^(m/2)
- Rationalizing denominators: √a / √b = √(a/b)
Statistics
- Mean: μ = Σ(x) / n
- Median: Middle value of a data set
- Mode: Most frequent value in a data set
- Range: Maximum value – Minimum value
- Standard deviation: σ = sqrt(Σ(x – μ)^2 / (n – 1))
Trigonometry
- Sine: sin(θ) = opposite / hypotenuse
- Cosine: cos(θ) = adjacent / hypotenuse
- Tangent: tan(θ) = opposite / adjacent
- Pythagorean theorem: a^2 + b^2 = c^2
- Law of sines: a / sin(A) = b / sin(B) = c / sin(C)
- Law of cosines: a^2 = b^2 + c^2 – 2bc cos(A)
Tables
Equivalent Forms of Linear Equations
| Form | Equation |
|---|---|
| Slope-intercept | y = mx + b |
| Point-slope | y – y1 = m(x – x1) |
| Standard | Ax + By = C |
| Horizontal | y = k |
| Vertical | x = h |
Rules of Exponents
| Rule | Expression |
|---|---|
| Product | a^m * a^n = a^(m + n) |
| Quotient | a^m / a^n = a^(m – n) |
| Power of a power | (a^m)^n = a^(mn) |
| Negative exponent | a^-n = 1/a^n |
| Zero exponent | a^0 = 1 |
Trigonometric Functions
| Function | Definition |
|---|---|
| Sine | sin(θ) = opposite / hypotenuse |
| Cosine | cos(θ) = adjacent / hypotenuse |
| Tangent | tan(θ) = opposite / adjacent |
| Cotangent | cot(θ) = 1 / tan(θ) |
| Secant | sec(θ) = 1 / cos(θ) |
| Cosecant | csc(θ) = 1 / sin(θ) |
Common Trigonometric Identities
| Identity | Formula |
|---|---|
| Pythagorean theorem | sin^2(θ) + cos^2(θ) = 1 |
| Double-angle formulas | sin(2θ) = 2 sin(θ) cos(θ), cos(2θ) = cos^2(θ) – sin^2(θ) |
| Half-angle formulas | sin(θ/2) = ±sqrt((1 – cos(θ)) / 2), cos(θ/2) = ±sqrt((1 + cos(θ)) / 2) |