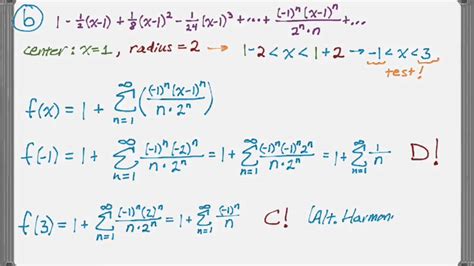In the realm of mathematics, Calculus BC and Calculus 2 stand as indispensable tools for delving into the complex world of calculus. These courses provide a foundation for understanding advanced concepts in science, engineering, and other disciplines.

Calculus BC
Calculus BC, an extension of Calculus AB, delves into more rigorous and advanced topics such as:
- Limits and Continuity: Exploring the behavior of functions as their inputs approach specific values.
- Derivatives: Investigating the rate of change of functions and their applications in optimization and related rates problems.
- Integrals: Studying the area under curves, volumes of solids of revolution, and other applications involving the accumulation of quantities.
- Applications of Calculus: Utilizing calculus to solve real-world problems in fields such as physics, economics, and biology.
Calculus 2
Building upon the concepts learned in Calculus BC, Calculus 2 introduces students to:
- Sequences and Series: Analyzing the limit behavior of sequences and determining the convergence or divergence of infinite series.
- Polar Coordinates: Describing points and curves in a plane using polar coordinates.
- Vectors and Functions in Space: Exploring vectors in three-dimensional space and their applications in physics and engineering.
- Partial Derivatives: Investigating functions with multiple variables and calculating their partial derivatives.
- Multiple Integrals: Extending the concept of integration to functions with multiple variables, enabling the calculation of volumes and surface areas.
Why Study Calculus BC & Calculus 2?
- Enhanced STEM Skills: Calculus BC and Calculus 2 strengthen analytical and problem-solving skills essential for success in STEM (science, technology, engineering, and mathematics) fields.
- Improved Critical Thinking: These courses foster critical thinking and the ability to analyze complex relationships and patterns.
- Higher Education Preparedness: Calculus BC and Calculus 2 serve as prerequisites for many advanced courses in science and engineering at universities.
- Career Opportunities: Proficiency in calculus opens doors to promising careers in various industries, including finance, technology, and data science.
- Personal Fulfillment: The intellectual challenge and satisfaction of mastering calculus can be immensely rewarding.
Common Mistakes to Avoid
- Lack of Practice: Regular practice is crucial for success in calculus. Skipping or rushing through exercises can lead to a shaky foundation.
- Overreliance on Calculators: While calculators can be helpful tools, over-reliance can hinder the development of conceptual understanding.
- Neglecting Graphing: Visualizing functions and their graphs provides valuable insights and helps identify patterns.
- Poor Note-Taking: Taking comprehensive notes during lectures and reading assignments ensures a solid reference for studying and review.
- Procrastination: Calculus is a sequential subject, and procrastination can lead to a snowball effect, making it difficult to catch up.
Strategies for Success
- Attend Classes Regularly: Engage actively in class discussions and seek clarification when needed.
- Complete Homework Assignments: Homework provides essential practice and feedback on your understanding.
- Form Study Groups: Collaborating with peers can enhance comprehension and provide different perspectives.
- Seek Help from Tutors or Professors: Don’t hesitate to ask for assistance when you encounter difficulties.
- Utilize Online Resources: Numerous websites and videos offer supplemental materials and practice questions.
Pain Points and Motivations for Learning Calculus BC & Calculus 2
Pain Points:
- Challenge: Calculus can be demanding and requires considerable effort and dedication.
- Uncertainty: Students may feel overwhelmed by the abstract nature of some concepts.
- Lack of Real-World Connections: Some students struggle to see the practical applications of calculus.
Motivations:
- Intellectual Curiosity: The satisfaction of understanding complex mathematical concepts drives many students.
- Career Aspirations: Calculus is a fundamental requirement for careers in STEM fields.
- Cognitive Improvement: Calculus enhances critical thinking and problem-solving skills, beneficial in various aspects of life.
- Educational Advancement: Calculus is essential for higher education in science and engineering.
- Personal Fulfillment: Mastering calculus provides a sense of accomplishment and academic achievement.
Applications of Calculus BC & Calculus 2 in the Real World
The applications of Calculus BC and Calculus 2 extend far beyond the classroom and into numerous fields, including:
- Physics: Modeling motion, forces, and energy conservation.
- Engineering: Designing structures, optimizing processes, and analyzing fluid flow.
- Economics: Predicting market trends, revenue optimization, and forecasting economic growth.
- Computer Science: Developing algorithms, modeling data, and optimizing software performance.
- Biomedicine: Analyzing medical imaging, modeling disease progression, and simulating drug delivery systems.
Innovative Applications: “CalcuThink”
To inspire creative thinking in calculus, we introduce the concept of “CalcuThink”: an approach that fosters the application of calculus to novel and unexplored domains. By embracing CalcuThink, students and practitioners can generate innovative ideas and solutions across diverse disciplines.
Tables for Calculus BC & Calculus 2
| Topic | Common Derivatives | Common Integrals | Applications |
|---|---|---|---|
| Limits and Continuity | $\lim_{x \to a} \frac{f(x)-f(a)}{x-a}$ | Indefinite: $\int f(x) \, dx$ | Convergence tests |
| Derivatives | Power rule, chain rule, product rule, quotient rule | Definite: $\int_a^b f(x) \, dx$ | Optimization, related rates |
| Integrals | Integration by substitution, integration by parts | Area under curves, volumes of solids | Work, fluid forces |
| Applications of Calculus | Rate of change, optimization, related rates | Length of curves, surface area | Modeling, real-world problem-solving |
| Topic | Sequences and Series | Vectors and Functions in Space | Partial Derivatives |
|---|---|---|---|
| Convergent Series | Geometric series, harmonic series, alternating series test | Vector operations | Directional derivatives |
| Divergent Series | p-series test, integral test | Vector functions, parametric equations | Gradient, divergence, curl |
| Sequences | Limit of a sequence | Surfaces, level curves | Chain rule for partial derivatives |
| Applications | Convergence tests, Taylor series | Motion along curves | Maxima and minima |
Conclusion
Calculus BC and Calculus 2 are essential courses for achieving academic success in STEM fields and beyond. By embracing a proactive learning approach, understanding common pitfalls, and exploring innovative applications through “CalcuThink,” students can not only master these courses but also develop invaluable critical thinking and problem-solving skills that will serve them well throughout their lives.