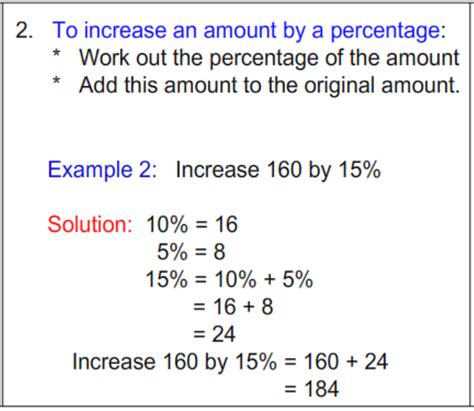
Understanding percentages is crucial for various aspects of life, from financial calculations to scientific measurements. In this article, we will focus on calculating and applying 10 percent of 260.

Calculating 10 Percent of 260
To calculate 10 percent of 260, you can use the formula:
Percentage (%) = (Part / Whole) * 100
Substituting the given values:
10% = (x / 260) * 100
Solving for x:
x = (10 * 260) / 100
x = 26
Therefore, 10 percent of 260 is 26.
Applications of 10 Percent of 260
Understanding 10 percent of 260 has practical applications in various domains:
Finance
- Calculate the down payment for a house: A 10% down payment on a $260,000 house would be $26,000.
- Determine sales tax: If the sales tax rate is 10%, a purchase of $260 would incur $26 in tax.
- Manage investments: Convert 10% of your portfolio into a higher-growth investment to optimize returns.
Engineering
- Calculate the margin of error: In engineering measurements, a 10% margin of error on a 260-meter measurement would be 26 meters.
- Determine safety factor: Factor in a 10% safety margin to ensure the strength of a 260-kilogram bridge design.
Manufacturing
- Control product quality: Set a tolerance of 10% for a product’s dimensions to ensure uniformity.
- Optimize production schedules: Adjust production schedules by 10% to accommodate unexpected demand or supply chain disruptions.
Customer Validation Through Questions
Understanding customer needs is essential for businesses. By asking the right questions, you can validate customers’ pain points, motivations, and desired outcomes:
- Pain points: “What challenges or frustrations do you face when…?**
- Motivations: “What are your primary reasons for using or considering…?**
- Desired outcomes: “What specific results or improvements would you like to achieve by using…?”
Tips and Tricks
- Use a calculator: Ensure accuracy by using a calculator for percentage calculations.
- Convert to decimals: Multiply the percentage by 0.01 to convert it into a decimal. This simplifies multiplication and division.
- Estimate first: Approximate the answer mentally before using a calculator to check your result.
- Consider the context: Understand the context of the problem before applying the percentage calculation to ensure accurate interpretation.
Conclusion
Calculating and applying 10 percent of 260 is a practical skill with diverse applications. By understanding the formula and its uses, you can navigate various scenarios effectively. Remember to validate customer needs and use tips and tricks to enhance your calculations and decision-making abilities.
Table 1: Percentage Conversion Table
| Percentage | Decimal |
|---|---|
| 10% | 0.1 |
| 20% | 0.2 |
| 30% | 0.3 |
| 40% | 0.4 |
| 50% | 0.5 |
| 60% | 0.6 |
| 70% | 0.7 |
| 80% | 0.8 |
| 90% | 0.9 |
| 100% | 1.0 |
Table 2: 10 Percent of Common Numbers
| Number | 10 Percent |
|---|---|
| 100 | 10 |
| 200 | 20 |
| 300 | 30 |
| 400 | 40 |
| 500 | 50 |
| 600 | 60 |
| 700 | 70 |
| 800 | 80 |
| 900 | 90 |
| 1000 | 100 |
Table 3: Applications of 10 Percent
| Domain | Application |
|---|---|
| Finance | Down payment, sales tax, investment strategy |
| Engineering | Margin of error, safety factor |
| Manufacturing | Product quality control, production schedule optimization |
Table 4: Tips and Tricks for Percentage Calculations
| Tip | Trick |
|---|---|
| Accuracy | Use a calculator |
| Decimals | Multiply percentage by 0.01 |
| Estimation | Approximate mentally |
| Context | Consider the problem details |